Modes of Fracture from Symmetry

In physics, we often use symmetry to break down a complicated linear problem into small pieces. The elastic deformations around a crack turn out to be a nice example of this method, with an interesting feature. Jennifer Hodgdon pointed out to me that we can understand Mode I, Mode II, and Mode III fracture using two symmetries of a straight crack front.

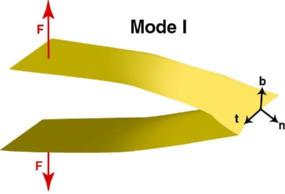
At the left, you see a growing crack. Although the crack can be curved in various ways, very close to the edge of the crack we can approximate it as a straight crack edge along a vector t, with a crack surface perpendicular to a vector b. The vector perpendicular to these two, pointing in the direction of crack growth, we call n.

There are two symmetries of the crack in this approximation.

• We can reflect the crack across the crack plane, giving an improper rotation R_b which takes b to -b and leaves n and t alone.
• We can reflect across the plane perpendicular to the crack edge, giving an improper rotation R_t which takes t to -t and leaves b and n alone.

Rb	Rt
Even	Even
Odd	Even
Odd	Odd

Any elastic deformation around the crack tip can be decomposed, using the principle of superposition, into four fields which are even or odd under the two symmetries R_b and R_t. This gives us the three classic modes of fracture. Mode I fracture, which pulls the crack open, is even under both symmetries. Mode II fracture, which shears the crack, is odd under R_b and even under R_t. Mode III fracture, which tears the crack in the third dimension, is odd under both R_b and R_t.

What about the deformation which is even under R_b and odd under R_t? If you think about it, this deformation doesn't produce a displacement at the crack surface: it's part of the general elastic deformation allowed in an uncracked material.

Links

Crack Growth Laws from Symmetry

Statistical Mechanics: Entropy, Order Parameters, and Complexity, now available at Oxford University Press (USA, Europe).