|
Basic forces on a concrete dam
note: a kip is a kilo-pound, equal to 1000 pounds (lbs.)
- forces of the reservoir water, Ww and Hw.
- Ww: vertical component of force of water -- weight of
water
Ww = (unit weight of water) * (volume of water)
the unit weight of water = 0.0624
kip/ft3 or 9810 N/m3
The weight of water should be disregarded for simulations.
- Hw: the horizontal component of the force of the
water
Water applies pressure (*) in the shape of
a triangle. The deeper the water, the more horizontal pressure it exerts on
the dam. So at the surface of the reservoir, the water is exerting no pressure
and at the bottom of the reservoir, the water is exerting maximum pressure.
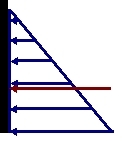 |
The base of the triangle is equal to the unit weight of water. The total
force exerted by the triangle equals the area of the triangle:
Hw = 0.5*(the
unit weight of water)*height2
height = the height of the
water
|
The force acts at the center of gravity of the triangle --
one-third of the way up from the bottom. The maroon arrow shows where the
total force of the water acts.
- uplift force, U: if there is no drainage, the uplift force is the
result of the water pressure under the dam pushing up on the dam
- weight of concrete, Wc
Wc = (the specific weight of concrete)*(volume of concrete)
the specific weight of concrete = 0.150 kips/ft3 or 23.6
N/m3
|
In a two-dimensional simulation, the thickness is assumed to be one. So the
volume is just equal to the area times 1. The weight is assumed to act at the
center of gravity (for simulation purposes, just make a guess at where the
center of the dam is and apply the weight there).
|
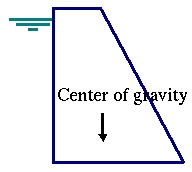 |


|




