We found a critical point in our model, separating smooth hysteresis loops from loops with a macroscopic jump or burst. At large disorder, every domain flips independently; at disorder small compared to the coupling between domains, a single domain flip can send its neighbors flipping until most of the system transforms in one burst. At the critical disorder, we get universal power laws and scaling.
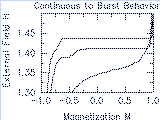
Phase transition as we vary disorder. Three H(M) curves for different levels of disorder: above, below, and near the critical disorderR_chen the burst disappears. (Select it for an animation!) For R > R_c the hysteresis loop is macroscopically smooth, although of course microscopically it is a sequence of sizable avalanches. The jump in the magnetization scales as (R-R_c)^beta, and the magnetization at R_c has a power-law form M - M_c ~ (H-H_c)^{1/delta}.
This is a simulation of a two-dimensional somewhat above the critical point, where none of the avalanches are very large.
This is a simulation closer to the transition. Notice the big avalanche which goes from one end of the system to the other! We're not sure yet whether there is a real transition in two dimensions: it's conceivable that no matter how weak the disorder, in a big enough system all avalanches would come to a halt at finite sizes. (We're sure that it happens that way in one dimension: there a single stuck spin can block the avalanche!)
Avalanches come in various sizes: Log-Log Plot of the avalanche-size distribution D(s) vs. avalanche size s, integrated over one sweep of the magnetic field from -infinity to +infinity, averaged over 5 systems of size 120^3. Notice the power-law region D(s) ~ s^{-(tau + sigma beta delta)} and the cutoff at s_{max} ~ (R-R_c)^{-1/sigma}.
The critical point is where an infinite avalanche first occurs. Naturally, near the critical point, some of the avalanches get rather large - indeed, one gets avalanches of all scales. At least in our model, one gets rather large avalanches even pretty far from the critical point: we believe the noise in hysteresis loops is due to this critical point.
Entertaining Science done at
LASSP.
James P. Sethna, sethna@lassp.cornell.edu
Statistical Mechanics: Entropy, Order Parameters, and Complexity,
now available at
Oxford University Press
(USA,
Europe).