What is it which distinguishes the hundreds of different states of matter? Why do we say that water and olive oil are in the same state (the liquid phase), while we say aluminum and (magnetized) iron are in different states? Through long experience, we've discovered that most phases differ in their symmetry.
Figure 2. Which is more symmetric?
The cube has many symmetries. It can be rotated by 90 degrees, 180 degrees, or
270 degrees about any of the three axes passing through the faces.
It can be rotated by 120 degrees or 240 degrees about the corners,
and by 180 degrees about an axis passing from the center through
any of the 12 edges. The sphere, though, can be rotated by any angle.
The sphere respects rotational invariance: all directions are equal.
The cube is an object which breaks rotational symmetry: once the cube
is there, some directions are more equal than others.
Consider figure 2, showing a cube and a sphere. Which is more symmetric? Clearly, the sphere has many more symmetries than the cube. One can rotate the cube by 90 degrees in various directions and not change its appearance, but one can rotate the sphere by any angle and keep it unchanged.
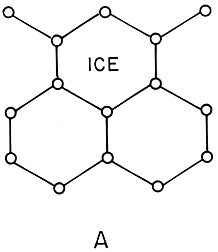
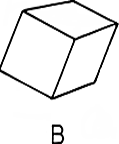
Figure 3. Which is more symmetric?
At first glance, water seems to have much less symmetry than ice.
The picture of ``two--dimensional'' ice clearly breaks the rotational
invariance: it can be rotated only by 120 degrees or 240 degrees
It also breaks the translational invariance: the crystal can only
be shifted by certain special distances (whole number of lattice units).
The picture of water has no symmetry at all: the atoms are
jumbled together with no long--range pattern at all. Water, though,
isn't a snapshot: it would be better to think of it as a combination
of all possible snapshots! Water has a complete rotational and translational
symmetry: the pictures will look the same if the container is tipped or
shoved.
In figure 3, we see a 2-D schematic representation of ice and water. Which state is more symmetric here? Naively, the ice looks much more symmetric: regular arrangements of atoms forming a lattice structure. The water looks irregular and disorganized. On the other hand, if one rotated figure 3B by an arbitrary angle, it would still look like water! Ice has broken rotational symmetry: one can rotate figure 3A only by multiples of 60 degrees. It also has a broken translational symmetry: it's easy to tell if the picture is shifted sideways, unless one shifts by a whole number of lattice units. While the snapshot of the water shown in the figure has no symmetries, water as a phase has complete rotational and translational symmetry.
One of the standard tricks to see if two materials differ by a symmetry is to try to change one into the other smoothly. Oil and water won't mix, but I think oil and alcohol do, and alcohol and water certainly do. By slowly adding more alcohol to oil, and then more water to the alcohol, one can smoothly interpolate between the two phases. If they had different symmetries, there must be a first point when mixing them when the symmetry changes, and it is usually easy to tell when that phase transition happens.
Jim Sethna, sethna@lassp.cornell.edu
Statistical Mechanics: Entropy, Order Parameters, and Complexity,
now available at
Oxford University Press
(USA,
Europe).