We introduce the theoretical framework we use to study the bewildering variety of phases in condensed-matter physics. We emphasize the importance of the breaking of symmetries, and develop the idea of an order parameter through several examples. We discuss elementary excitations and the topological theory of defects.
Paper published originally in 1991 Lectures in Complex Systems, Eds. L. Nagel and D. Stein, Santa Fe Institute Studies in the Sciences of Complexity, Proc. Vol. XV, Addison-Wesley, 1992.
As a kid in elementary school, I was taught that there were three states of matter: solid, liquid, and gas. The ancients thought that there were four: earth, water, air, and fire, which was considered sheer superstition. In junior high, I remember reading a book called The Seven States of Matter. At least one was ``plasma'', which made up stars and thus most of the universe, and which sounded rather like fire to me.
The original three, by now, have become multitudes. In important and precise ways, magnets are a distinct form of matter. Metals are different from insulators. Superconductors and superfluids are striking new states of matter. The liquid crystal in your wristwatch is one of a huge family of different liquid crystalline states of matter [1] (nematic, cholesteric, blue phase I, II, and blue fog, smectic A, B, C, C*, D, I, ...). There are over 200 qualitatively different types of crystals, not to mention the quasicrystals (figure 1). There are disordered states of matter like spin glasses, and states like the fractional quantum hall effect with excitations of charge e/3 (like quarks). Particle physicists tell us that the vacuum we live within has in the past been in quite different states: in the last vacuum but one, there were four different kinds of light [2] (mediated by what is now the photon, the W+, the W-, and the Z particle). We'll discuss this more in lecture two.
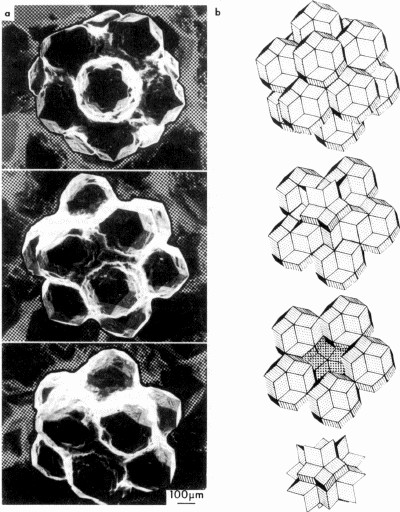
Figure 1. Quasicrystals.
Much of these two chapters will discuss the properties of crystals.
Crystals are surely the oldest known of the broken-symmetry phases of matter,
and remain the most beautiful illustrations. It's amazing that in the
past few years, we've uncovered an entirely new class of crystals. Shown
here is a photograph of a quasicrystalline metallic alloy, with
icosahedral symmetry. Notice the five-pointed stars: our old notions of
crystals had to be completely revised to include this type of symmetry.
Photograph courtesy of Marc Audier, Ecole Nationale Superieure
d'Electrochimie et d'Electrametallargie de Grenoble. Published
in J. H. Lang, M. Audier, B. Dubost, and P. Sainfort,
J. of Crystal Growth, 83, 456 (1987).
When there were only three states of matter, we could learn about each one and then turn back to learning long division. Now that there are multitudes, though, we've had to develop a system. Our system is constantly being extended and modified, because we keep finding new phases which don't fit into the old frameworks. It's amazing how the 500th new state of matter somehow screws up a system which worked fine for the first 499. Quasicrystals, the fractional quantum hall effect, and spin glasses all really stretched our minds until (1) we understood why they behaved the way they did, and (2) we understood how they fit into the general framework.
In this lecture, I'm going to tell you the system. In the next lectures, I'll discuss some gaps in the system: materials and types of behavior which don't fit into the neat framework presented here. I'll try to maximize the number of pictures and minimize the number of formulas, but there are problems and ideas that I don't understand well enough to explain simply. Most of what I tell you in this lecture is both true and important. Much of what is contained in the next lectures represents my own pet ideas and theories, and you should be warned not to take my messages there as gospel.
The system consists of four basic steps.[3] First, you must identify the broken symmetry. Second, you must define an order parameter. Third, you are told to examine the elementary excitations. Fourth, you classify the topological defects. Most of what I say I take from Mermin[3], Coleman[2], and deGennes[1], and I heartily recommend these excellent articles to my audience. We take each step in turn.
Jim Sethna, sethna@lassp.cornell.edu
Statistical Mechanics: Entropy, Order Parameters, and Complexity,
now available at
Oxford University Press
(USA,
Europe).