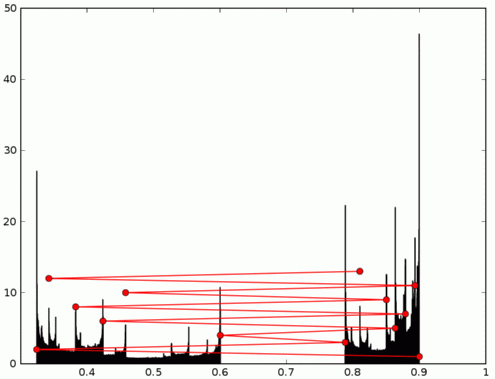
Chaotic dynamical systems will stretch and fold the state space. In
Hamiltonian systems, this stretching and folding preserves volume in
phase space, and the natural weighting of all points in phase space
is equal (Liouville's theorem). In more general chaotic dynamical systems with
dissipation and forcing, the long-time behavior lies on an attractor:
points off of the attractor do not contribute to the time-average behavior,
and points on the attractor are weighted by an invariant measure
or probability density in state space.
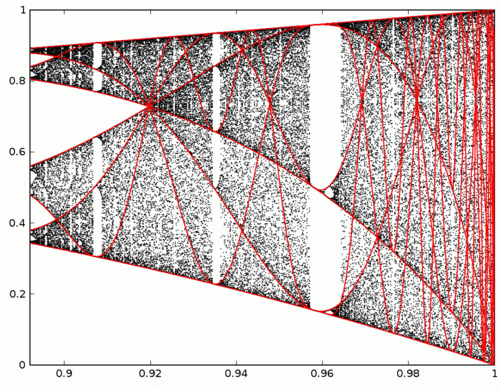
In this problem we explore the invariant measure for the logistic map,
which goes chaotic through a
period doubling cascade. The chaotic region of this map exhibits
a complex, textured structure with a series of sharp internal boundaries.
In this exercise, we learn to understand the
Last modified: August 24, 2006
Statistical Mechanics: Entropy, Order Parameters, and Complexity,
now available at
Oxford University Press
(USA,
Europe).