H2: Single Slit Diffraction

We now explore the diffraction pattern from a single slit of width a. Load the preset SngDiff. Notice the complicated pattern in the wave tank. Notice on the screen far away, there is a peak which goes almost to zero, then has secondary peaks on either side.

(H2a) The minima.

Measure the position of the first minimum. Include it in your write-up.

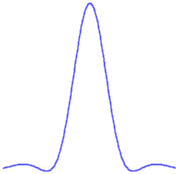
At this minimum, the phases from the different portions of the slit must cancel. Since the slit produces a continuous range of phases, the contributions will cancel out if all phases are weighted equally. (The average of sin(x + phi) when phi varies over two pi is zero.) This happens if the two ends of the slit have phases which differ by 2 pi: that is, the condition that a slit of width a has a minimum is that the two ends of the slit interfere constructively. (Isn't that perverse?) Give the formula for the angle of this first minimum, without using the small-angle approximation. Calculate the distance you predict for the minimum, and compare with your prediction.

Notice that the minima in the pattern are roughly equally spaced, except that the central peak is twice the width of the others. If the phase from the slit varies by 2 m pi over the width of the slit, then all phases are weighted equally. Explain in your write-up why is this not true for m=0, the central peak.

Make the wavelength larger. At Lambda=1, use Screen size to increase the screen size to 300m, and use configure... to change Wave-tank Size L to 30. Can you see the beams emitted corresponding to the central and side peaks? For Lambda=10, how different is the pattern on the screen for a=1 from that for a=0?

(H2b) Small wavelengths and geometrical optics.

You normally don't see diffraction patterns when you look out the window! Reload the preset SngDiff. Notice the waves look almost like a beam, with ratty edges. Reduce the wavelength Lambda to 0.2. Notice the ratty edges become much less prominent. (You can play with Wave-tank resolution and Color Saturation Amp to get better pictures, especially if you have a fast machine.) In your write up, describe the waves in the wave tank when the wavelength is small compared to the slit width. Light travels in straight lines only because the wavelength is short compared to the width of the window!

Geometrical optics, which is the science which allowed us to design lenses, microscopes, and telescopes, is precisely the ``straight-line'' behavior of waves in vacuum when they have very short wavelengths compared to the obstacles in their path. Geometrical optics ignores diffraction, and treats light as being composed of rays.

Links Back

Statistical Mechanics: Entropy, Order Parameters, and Complexity, now available at Oxford University Press (USA, Europe).