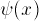Introduction to the simulation ``schrdgr''

Try moving the Energy slider at the top, and see the wave-function change! This is the quantum-mechanical wave function

for an electron in a potential, with definite energy given by the slider value. In this lab, we will be finding solutions of definite energy which satisfy the correct boundary conditions on the right. We'll do this for three different potentials: the infinite square well, the square-shelf potential, and the Coulomb potential for the hydrogen atom.

Shown are the three forms of V(x) we will study in this computer lab.

What equation are we solving? We are solving the time-independent Schrödinger equation for an electron in a one dimensional potential V(x):
.
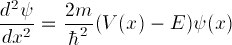Here Psi is the wave function, describing the state of the electron; x is position in space, m is the mass of the electron, is Planck's constant h divided by two pi, and V(x) is a potential energy well. We will be varying the energy E and looking for well-behaved solutions.
This is a special form of the general, time dependent Schrödinger equation
,
suitable for calculating solutions with a particular energy. In quantum mechanics, an electron can (and often is) in a state without a fixed energy (just like it doesn't have a fixed position): the time-dependent equation, like the equation for waves on a string, describes the evolution of the wave function. The time-dependent equation is very much like our wave equation (and often is called a wave equation). Notice that it involves i, the square root of minus one: we've been avoiding complex numbers in this course, and by specializing to the time-independent equation we can continue avoiding them. Also, the time-independent equation is simpler to solve: more like the pendulum than the wave equation. 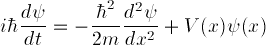Indeed, it's very much like the pendulum: the second derivative is proportional to the wave function 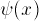, just like the second derivative of theta with respect to t was proportional to theta.
What do the solutions mean? Notice we aren't explaining here how one derives the time-independent equation from the time-dependent one: it's a bit complex. We'll just say that the time-independent equation describes solutions of fixed energy E: electrons in a fixed energy state keep the same wave-function, oscillating in phase (the complex number we keep ignoring). Solving the time-independent solution is like solving for the standing waves on a string: it doesn't describe all the behavior (like traveling pulses!) but it gives an excellent description of resonances and frequency dependence.
Many of you are familiar with electron wave-functions from chemistry. There one describes, for example, s and p states of various shapes, which can be occupied by the electron, binding it to the nucleus. The shapes of these wave-functions in large part determines the chemical bonding of the atoms. The energies of these wave-functions determines the frequencies of the light they can absorb and emit. Unlike waves on a string, each solution does not separately resonate with light of a particular frequency. Instead, light is emitted and absorbed when the electron changes from one state to another, and the frequency of the light is determined by the difference in energies between the two solutions:
frequency = (E2-E1)/
In this lab, we'll be determining the energies and shapes of the solutions.
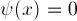How are we finding the solutions? Solving the time-independent equation is easy, once you know the boundary conditions and the energy - we use exactly the same method we used to solve the pendulum's motion. However, for the pendulum, both initial conditions (position Theta and velocity ThetaDot) were known at the initial time. For us, we know that at X=0, and (depending on the potential) either 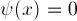at X=Xshelf, or 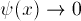as X goes to infinity. Because our boundary conditions involve two different points, this is an example of a two-point boundary value problem.
We'll solve the problem by "shooting": we pretend we know the derivative of 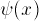at X=0, solve the equation, and then fiddle around until it has the right value at the other boundary. For our problem, we'll fiddle around with the energy E: raising and lowering the energy will change how the electron wave function acts at the right-hand boundary.
The default potential is a square well, with infinite walls. Try varying the energy to make the wave-function zero at the right-hand side! You'll find there are several solutions, with different numbers of nodes, just like there are several different shapes of wave-functions for electrons in your chemistry class.
Links Back
Statistical Mechanics: Entropy, Order Parameters, and Complexity, now available at Oxford University Press (USA, Europe).

Introduction to the simulation ``schrdgr''

Links Back